- Introductory (why, why why)
- Specific calculations
- Philosophical and practical part.
1. Introductory (why, why why)
The following circumstance pushed me to this post. There are a lot of materials on this subject in the internet. However, 80% of them suffer from a tragic flaw - they explain in sufficient detail why classical logic is wrong and confine themselves to the fact that in quantum mechanics everything is different and the observations correspond to the values predicted by it. The remaining part is loaded with a mathematical apparatus and after a string of formulas says - well, you see, it will be like that. After that, there is a sensation of pain from rape of the brain, because the real
And I want to understand, because in my deep conviction, nevertheless, the next revolutionary leap in technology will be associated with the mastery of quantum power by humanity. And just as in the 60s people couldn’t even imagine how these huge glands occupying entire cellars would change our lives, drastically reducing their size and increasing power, and now we do not imagine the full potential of clumsy prototypes with 50 qubits.
Fortunately, however, there are really cool dudes who are trying to convey the physical meaning of quantum phenomena. But perhaps precisely because of their coolness, such professional explanations turn out to be unnecessarily complicated. I think this is largely due to the fact that the pros are psychologically difficult to say, for example, that the spin of entangled electrons is the same. Whereas in reality it is the opposite. However, it is difficult for a person not in the subject to keep such aspects in mind, as a result, after the next sketch of terms and a portion of formulas, the brain simply boils and filters the information that comes next.
But this is not so bad. About three years ago, I sat down and sweated a lot, figured out how it works and calmed down on this. But recently, with surprise, I discovered that when I tried to remember what was happening, I found a gaping void in my head. The conclusions that come from the analysis are so counterintuitive that they don’t fit in your head. Therefore, having crossed this path anew, I decided to write down a chain of reasoning in the simplest, but at the same time absolutely understandable (at least to me) way. I hope this is useful not only to me, but the conclusions that follow from this phenomenon, in my opinion, are quite interesting.
Well, ending the introduction, I think it makes sense to say that I will simplify the description as much as possible, but not to the detriment of the essence of quantum phenomena. It may be difficult to believe in this, but it turns out that you can do with just one formula, and even then, it is not required by and large.
2. Specific calculations
So let's go. Suppose we have an atom that emits two electrons scattering in opposite directions arbitrarily far, for example, into different galaxies. Suppose each electron in a pair has the same spin (angular momentum). In fact, the backs are opposite, but for simplicity we will assume that they are equal, since this does not affect the final result.
After the measurement, the spin may turn up or down relative to the measuring device (P). Moreover, different pairs have their own spin orientations, which are not connected in any way with other pairs.
For example, the first radiation of an atom generated a pair of electrons (e) and we set the device at an angle of 0 degrees. Then at the exit, after the device, we find them in a state up or down. We do not know where exactly (here 50/50), but it will be exactly the same. In the figure below, the blue state is up.
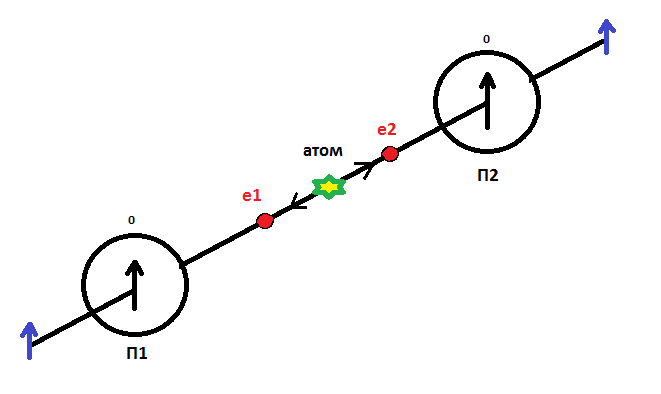
Fig. one.
If you try to measure these electrons along any other axis, for example 120 degrees, the electrons will also be detected, they creep up this direction or down. In this case, suppose they go down.
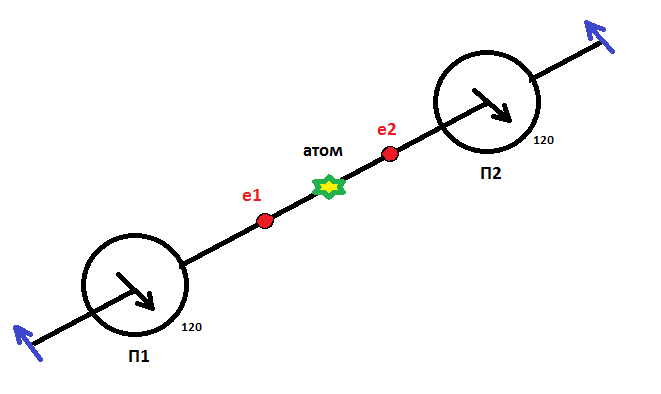
Fig. 2.
It is worth noting that “down” we call the direction of the reverse orientation of the device. And here a key question pops up, but how do the electrons decide in which direction they will go, up or down?
The usual way of thinking tells us - regardless. They “know” in advance in which direction to go one or another device and after scattering do not affect each other in any way. This is called the theory of hidden parameters. Those. it implies that we do not know how they solve it, but since the result of their choice is always exactly the same, there are such parameters, we are just a little dumb and have not yet caught up with what they are.
Quantum mechanics, however, tells us that operations on a single particle instantly affect the state of entanglement with it, even if there are billions of light years between them. There, Einstein and his comrades were specifically bombed about this, but not the point. And as we will see below, there is a really simple and understandable description of this phenomenon. However, before moving on to it, let's understand how classical logic breaks its rotten teeth into true reality.
To do this, we will do a simple thing, begin to measure the spins of electrons, spinning the instruments, independently of each other in three different directions: at an angle of 0, 120 and 240 degrees. Those. for example, P1 will be installed in one of three positions completely by accident. This can be determined, for example, by throwing a cube.
- If 1 or 2 falls out, then there will be a position of 0 degrees.
- If 3 or 4 falls out, then it is 120 degrees.
- If 5 or 6 drops out, this is 240 respectively.
Also for P2, another die will be thrown and, depending on the result of the throw, the second device will be installed in one of three positions.
As we saw earlier, if the devices accidentally stand in the same direction, the results will always be the same and therefore this case is not interesting to us. Another thing is if the devices stand in different positions, for example, like this:

Fig. 3.
Here we see that P1 stood at an angle of 120 degrees and e1 passed through it with its back up. And P2 was at an angle of 240 and e2 went with his back down. As already mentioned, we assume that the electrons carry the same program of passage of devices. And here it will be useful to write out all the possible combinations for each electron:

Table 1.
By the word program, we mean the very hidden parameters that determine how the electron passes through the device. For example, Figure 3 implements program 3 or 7. We do not know exactly which one, because it is not clear how the electrons would behave when passing through a device set to 0 degrees.
So the prediction of our ordinary logic, which will be refuted, is extremely simple. If we analyze only those cases when the directions of the instruments do not coincide, then the directions of the spins e1 and e2 after passing through the instruments will coincide exactly in half the cases. Let's make sure of this by painting all the combinations in the form of a tablet:

Table 2.
Here, mirror cases such as “e1 by 0, e2 by 120” and “e1 by 120, e2 by 0” are combined, since their programs are the same anyway.
The first program is not interesting, they always look down, because the coincidence of orientations is guaranteed. But the second program is more complicated. Here in one case there will be a coincidence when e1 goes through angle 0 and looks down, and e2 goes through angle 120 and also looks down. But already if e2 passes through an angle of 240, then it will look up (this is its internal parameters in this program) and then there will be no coincidence.
It is easy to verify that the number of matches is 12, i.e. exactly half the cases. This is logical, understandable and, as the experiment proves, is completely wrong. The fact is that there will be only 6 matches. And looking at the plate above it is impossible to understand how this can happen.
Here, at best, the reader is offered something like this:

Fig. 4
Seriously, you can figure out these sconces and ketos, but what will it give? We still won’t have a real understanding of what happened, but we want to understand the essence of the phenomenon.
But the essence is as follows. Let us consider a little more carefully the flight of the first electron. Suppose he faces a severe test with a device installed at an angle of 0. The electron has a certain moment of momentum and depends on its direction, what will happen when the device passes. Suppose it is 80 degrees. Then the electron with a probability of 0.58 selects the state up and with a probability of 0.42 state down. Why exactly - so far no one is able to answer. All that humanity has so far been able to do is find a magic formula that predicts this and looks like this:
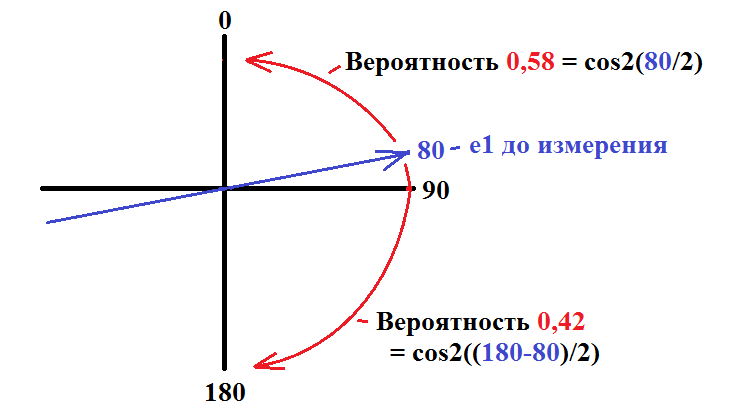
Fig. 5
Those. we take the angle between our angular momentum of 80 and 0 (in our case it turns out to be 80), divide by 2 and substitute it into the square cosine and you're done. As far as I understand, they found this formula empirically, because put the devices, shot, looked at the results, then figured out what function describes the result, and please. But if this is not the case and someone knows how it is displayed, then it will be very interesting to know this and welcome to the comments.
And here is the good news, friends will rejoice, there will be no new formulas, this is the first and last. Therefore, it should be recognized, otherwise it will not be very clear further. So, let’s say that our particular electron chose to pass the device upward (it could be down, but the probability of this is less, as we see).
And here is the most interesting thing that will happen to the second electron? Which after a moment flies up to his device, installed at an angle of 120 degrees. If these inhuman experiments were not performed with the first electron, then the internal angular momentum of the second would be equal to 80. And then the probability of choosing an upward state for angle 120 would be equal to 0.88:
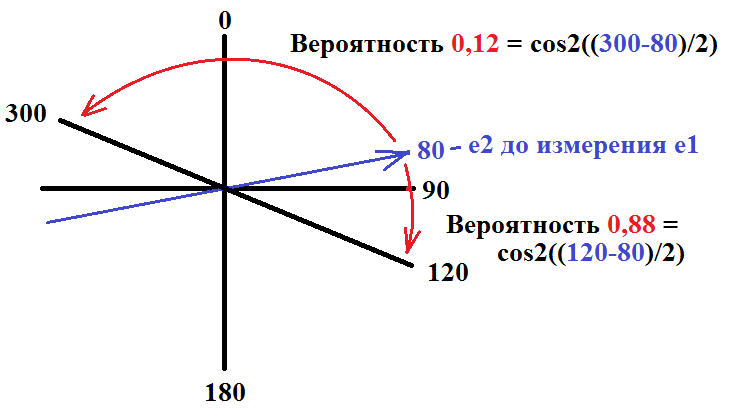
Fig. 6
But then quantum magic begins to work. The second electron instantly “learns” that its distant fellow has just made a choice upward relative to an angle of 0 degrees. And now he behaves when passing the device 120 degrees accordingly:
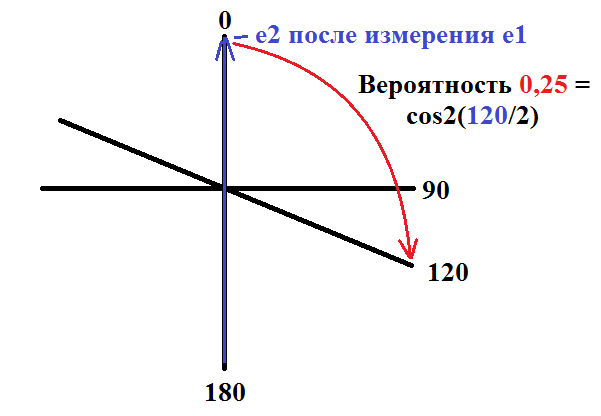
Fig. 7
Those. the inner spin of e2 abruptly changed from 80 to 0, because somewhere in another galaxy, someone threw the die just like that and put the device in front of e1 by 0 degrees!
Accordingly, the probability of e2 passing through the device 120 degrees with the state up decreases from 0.88 to 0.25. That is why the circuit described in table 2 breaks.
Now we can easily understand why instead of 12 matches, according to Table 2, there will only be 6. If before, before exposure to P1 on e1, the probability of passing e2 through P2 by one or another state was on average 0.5, now it has become strictly 0.25. Accordingly, the number of matches will fall by 2 times.
The classical world with programs wired into electrons is defeated, the incomprehensible connection between the objects infinitely distant from each other triumphs.
3. The philosophical and practical part.
Now, realizing the reality of what has been proved in paragraph 2. fact, it’s interesting to think about such things. Entangled states are very common in nature. For example, atom decays give rise to entangled states of scattering particles, they cling to others and, ultimately, interact with us.
And so, just out of a whim, clicking my fingers here, I instantly change the state of a huge number of entangled particles scattered throughout the universe. And since most processes in nature are unsteady (otherwise we would not have observed its evolution), this means the launch of a whole cascade of events that radically change the world around us.
This is not very often seen in real life, because we have nothing to compare with, before our eyes there is always one version of reality that has been realized. However, when I was engaged in modeling economic systems, it was interesting to observe how the change of even one bit, among millions of others, led to the fact that the evolution of the whole world went in a completely different way. Yes, in general, the system still developed within certain limits, the aggregates of this did not change much, but the individual fate of the participants changed dramatically, after a very short time.
This is the same butterfly effect, which seems to us more about insects than about us, is repeatedly enhanced by quantum entanglement. It's funny, but it turns out that just sneezing can cause an eruption of a volcano in another galaxy.
But, unfortunately, it is not yet clear how to manage this more consciously, so it is interesting to mention the applied aspects of this phenomenon. In the picture below, one of the first Cray-1 supercomputers with a performance of 133 MFlops.

Modern smartphones that we carry in our pockets have a thousand times higher performance, about 150 GFlops. In this regard, there is a feeling that in 30 years we will be something similar in relation to quantum computing. Yes, so far our fantasy is enough except for a quick search for a full name by phone number (Grover's algorithm) or hacking of encryption keys. But after all, in the 70s, few understood the degree of penetration of IT into our lives.
It seems to me that understanding the principles underlying quantum phenomena will make it easier to accept and harness the potential of the coming era of the quantum era.