How to Catch Light with Foam: Foam-Photon Network

Back in 1887, the Scottish physicist William Thomson proposed his geometric model of the structure of the ether, which supposedly was an all-pervasive medium, the vibrations of which appear to us like electromagnetic waves, including light. Despite the complete failure of the theory of ether, the geometric model continued to exist, and in 1993 Denis Weir and Robert Phelan proposed a more advanced model of the structure that could fill the space as much as possible. Since then, this model has been mostly interested in mathematicians or artists, but recent research has shown that it can become the basis for future technologies that use light instead of electricity. What is the Weyr-Phelan foam, what is its unusualness and how can it be used to capture the light? We will find answers to these and other questions in the report of the research group. Go.

Study basis

Just a hundred years ago, in the scientific community there was a very curious theory about some kind of matter all around. This theory was aimed at explaining the nature of electromagnetic waves. It was believed that ether surrounds everything and is the source of these waves. The scientific discoveries that followed the theory of ether completely destroyed it.





William Thomson



However, in 1887, when the theory of ether was full of strength and popularity, many scientists expressed their ideas on how exactly ether can fill the whole space. William Thomson, also known as Lord Kelvin, was no exception. He was looking for a structure that would ideally fill the space so that there were no empty areas. These searches were later called the “Kelvin task”.



A primitive example: imagine a box containing cans of cola. Between them, due to the cylindrical shape, voids arise, i.e. unused space.



Thomson, in addition to the belief that the Earth is no more than 40 million years old, proposed a new geometric structure, which was improved by Denis Weir and Robert Phelan, and therefore was named in their honor.



The Weir-Phelan structure is based on cells that fill the space with disjoint polyhedra, and there is no empty space. Honeycombs, which we usually represent in the form of hexagons due to bee honeycombs, actually come in different shapes. There are cubic, octahedral, tetrahedral, rhombic dodecahedral, etc.





Weir Phelan Structure



The peculiarity of the Weir-Phelan honeycomb is that they consist of different geometric shapes. At its core, it is an ideal foam from bubbles of the same size.



The ancestor of this foam was that proposed by Lord Kelvin, already familiar to us. However, its variant consisted of shortened cubic honeycombs. The Kelvin structure was a convex homogeneous honeycomb formed by a truncated octahedron, which is a polyhedron with four faces, filling the space (tetradecahedron), with 6 square faces and 8 hexagonal faces.



This option of maximally filling the space was considered ideal for almost a hundred years, until in 1993 Weir and Phelan opened their structure.





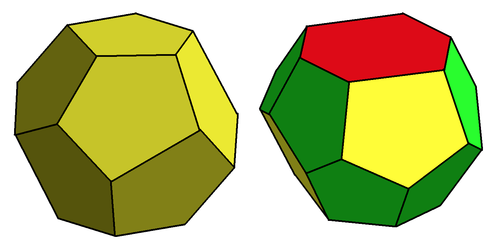
Pentagon and decahedron



The main difference between the Weir-Phelan cell and its predecessor is the use of two types of constituent elements, which, nevertheless, have the same volume: a pentagon-dodecahedron (a tetrahedron with tetrahedral symmetry) and a tetrahedron with rotational symmetry.



In the work we are considering today, scientists from Princeton University decided to use Weir-Phelan foam in photonics. First of all, it was necessary to find out whether photonic band gaps (PBGs) of such a foam block light propagation in all directions and for all polarizations in a wide frequency range.



In their study, scientists demonstrated that a three-dimensional photon network based on the Weir-Phelan foam leads to significant PBG (16.9%) with a high degree of isotropy * , which is an important property for photon chains.

Isotropy * - the same physical properties in all directions.

Kelvin foam and C15 foam also showed good PBG results, but they are inferior to the Weir-Phelan structure in this indicator.



Similar studies were previously conducted, however, they focused on two-dimensional dry foam. Then it was found that two-dimensional amorphous dry foam exhibits PBG only for transverse electric polarization. The problem is that there are two polarizations in three-dimensional foam.



Despite possible difficulties, three-dimensional foam can be safely considered a promising material in the field of photonics, according to the researchers. There is a reason for this: the laws of the Plateau guarantee that the edges form exclusively tetrahedral vertices. And this is a big plus for photonic networks. A vivid example of this is a diamond with a PBG of 30%.



Foam has the tetrahedral property of the coordinates of the diamond lattice, but they differ in that they have curved edges and somewhat different lengths of bonds. It remains only to find out how and to what extent such differences affect the photonic properties.



If the ribs of three-dimensional dry foam are made thicker, then photon networks can be created (images below), which show pronounced photonic PBGs of up to 17%, comparable or superior to those in typical examples of self-organizing photonic crystals.





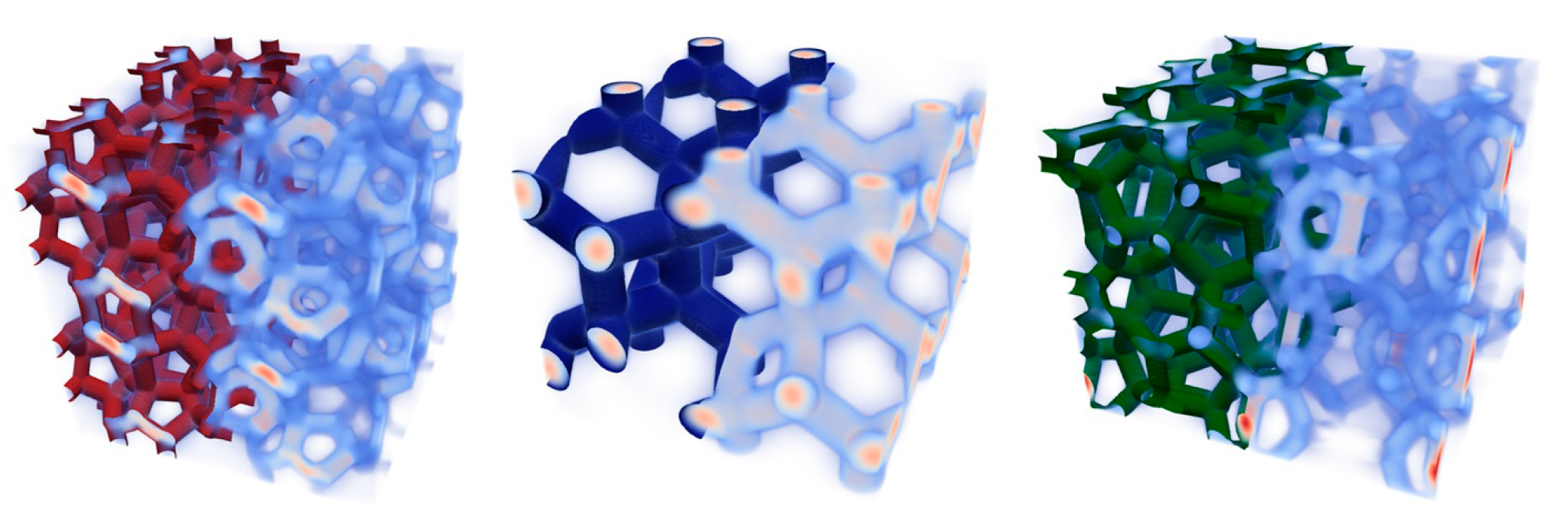
Image No. 1: photon-foam networks obtained by thickening the edges of the Weir-Felan structure (left), Kelvin structure (center) and C15 foam (right).



In order to implement such a model in practice, dry foam must first be crystallized and then coated with a dielectric material. Naturally, the PBG of the foam will be less than that of the photonic crystal, but this disadvantage can be overcome by a number of advantages. First, the self-organization of the foam can allow the rapid production of large samples. Second, photon-foam-based heterostructures, taking into account previous studies, can have a wider range of applications.

Research results

First of all, it was necessary to study dry foam, which is defined as local minima of the tessellation interphase * taking into account volume restrictions, so that the final geometry obeys the Plateau laws.

Tessellation * - breaking a plane into components that completely cover the entire plane without leaving spaces.

To build the Weir-Phelan, Kelvin, and C15 foams, scientists began with weighted Voronoi tessellations for BCC, A15, or C15 crystals, respectively.





Voronoi diagram



The parameters were chosen so that all separation cells had the same volume.



Nets formed from curved ribs of foams and from straight ribs of the tessellations of their predecessors were studied. To evaluate the topology of all types of foam, ring statistics * were used.





In the case of the study in question, the number of shortest rings per vertex in the unit cell was calculated.



One cell in the Kelvin model has 2 squares and 4 hexagons per vertex, but TCP (tetrahedrally tightly packed) foam has only pentagonal and hexagonal faces (average values: 5.2 and 0.78 in Weir-Felan foam; 5.3 and 0.71 in C15 foam). Voronoi tessellations A15 and C15 are TCP structures with the largest and smallest number of faces ( f ) per cell. Thus, the Weir-Phelan structure has the largest number of faces ( f = 13 + 1/2), and C15 has the smallest number of faces ( f = 13 + 1/3).



Having finished with theoretical training, the scientists started modeling the photon network based on the edges of dry foam, i.e. foam-photon network. It was found that with a PBG value of 20%, the characteristics of the system are maximized, and at 15%, the Weir-Phelan foam becomes unstable. For this reason, scientists did not consider wet foam, where the boundaries of the Plateau have tricuspoid sections. Instead, all attention was focused on dry foam structures, where scientists could gradually increase the thickness of the ribs.



In addition, each rib is the medial axis of the spherical cylinder (capsule), where the radius is a setting parameter.



Researchers recall that such foam networks are not literally foam, but in their report they will be referred to as “foam” or “foam network” for ease of storytelling.



During the simulation, the parameter ɛ (dielectric contrast) was taken into account - the proportion of dielectric constants of materials with high and low insulation values. It is assumed that the dielectric contrast is from 13 to 1, which is usually used in the literature as a standard when comparing the characteristics of various structures of photonic materials.



For each network, the radius of the ribs (spherical cylinders) is optimized for the maximum ratio of the forbidden band and its middle: ∆ ω / ω _m , where ∆ ω is the frequency band width and ω _m is the frequency inside the band.





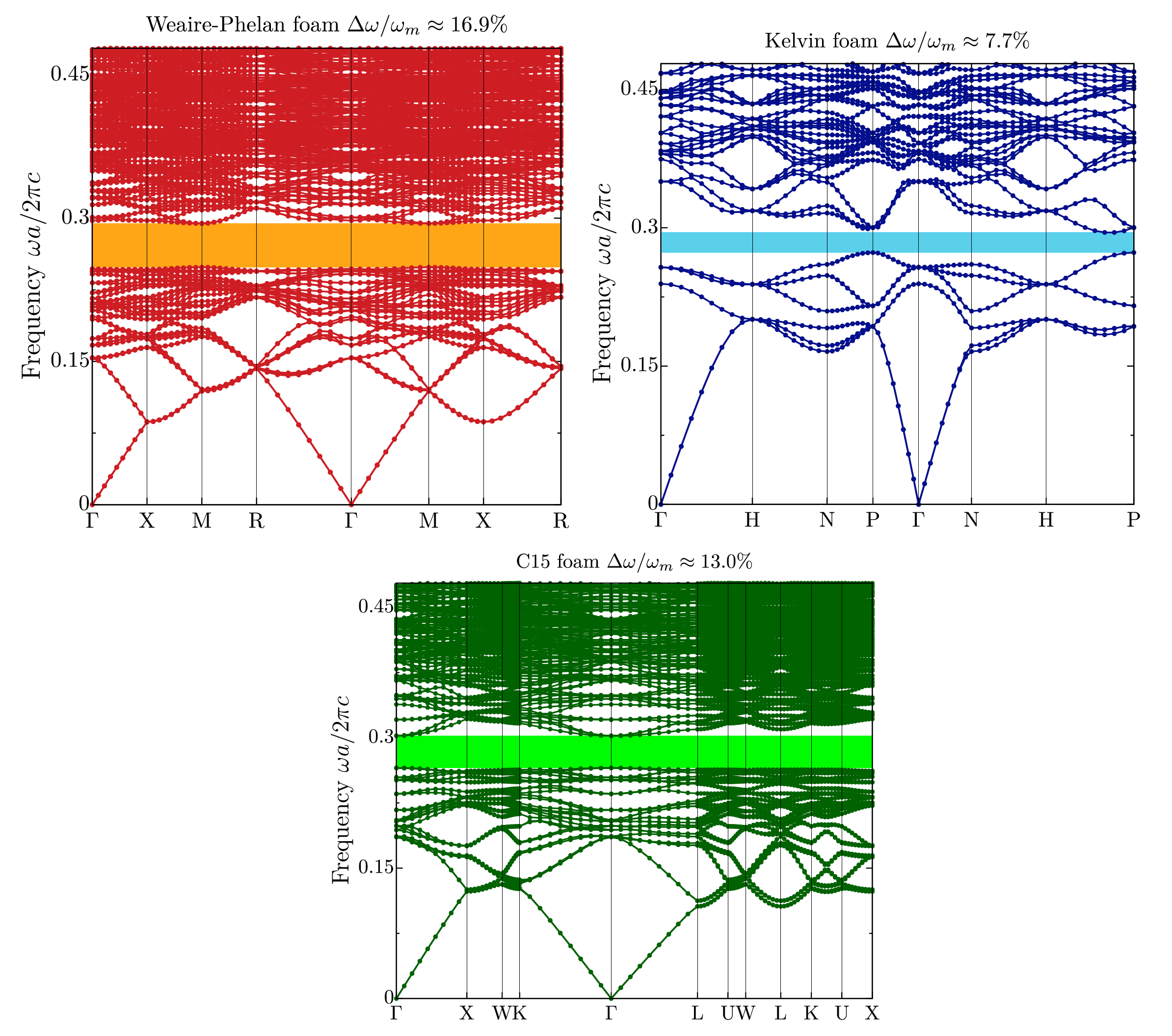
Image No. 2: photon zonal structure of Weir-Felan foam (red), Kelvin foam (blue) and C15 foam (green).



Next, PBG sizes were measured, which were: 7.7% for Kelvin foam, 13.0% for C15 foam, and 16.9% for Weir-Felan foam. Area minimization increases PBG sizes by 0.7%, 0.3, or 1.3%.



As it became clear from the analysis, TCP networks have much larger PBG sizes than Kelvin networks. Of the two TCP networks, it is the Weir-Felan foam that has the largest size of the forbidden zones, which, presumably, is associated with a smaller change in the length of the bonds. Scientists believe that differences in bond lengths may be the main reason why in their system, i.e. in Weir-Phelan foam, PBG is lower than in diamond (31.6%) or in the Laves system (28.3%).



An equally important aspect in photonics is the isotropy of PBG, which allows the creation of arbitrary waveguides. Photonic quasicrystals, as well as amorphous photonic networks, are more isotropic than classical photonic crystals.



The studied foam-photon structure also has a high degree of isotropy. Below is the formula for determining the anisotropy coefficient (i.e., the degree of difference in the properties of a particular medium) PBG ( A ):



A: = (√ Var [ ω _HDB ] + Var [ ω _LAB ]) / ω _m



It was found that the C15 foam has the lowest anisotropy (1.0%), followed by the Weyr-Felan foam (1.2%). Therefore, these structures are highly isotropic.



But the Kelvin structure demonstrates an anisotropy coefficient of 3.5%, which is quite close to the Laves index (3.4%) and diamond (4.2%). However, even these indicators are not the worst, because there are still simple cubic systems with an anisotropy coefficient of 8.8% and hexagonal diamond networks with 9.7%.



In practice, when it is necessary to reach the maximum value of PBG, sometimes it is necessary to change certain physical parameters of the structure. In this case, this parameter is the radius of the spherical cylinders. Scientists performed mathematical calculations in which they found out the ratio of the photonic band gap and its width in the form of the function ɛ . For each obtained value, radius optimization was performed to maximize ∆ ω / ω _m .





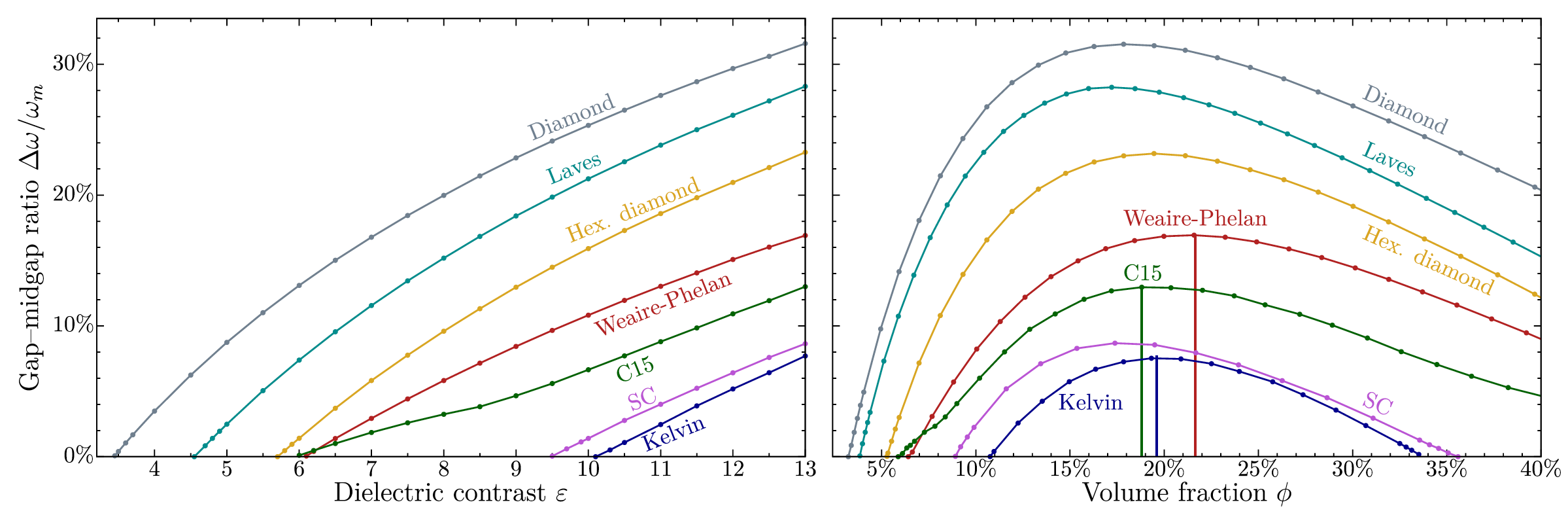
Image No. 3: Comparison ∆ω / ω _{m of the} investigated foam networks (C15, Kelvin, Weir-Phelan) and other structures (diamond, hexagonal diamond, Laves, SC - ordinary cubic).



Weir-Phelan foam maintains an acceptable PBG size of 8% up to a dielectric contrast of ɛ ≈9, and the radius has been increased to reach a maximum PBG of 15%. PBG disappear at ɛ <6.5. As expected, the diamond structure has the largest PBG of all the structures studied.



For a more detailed acquaintance with the nuances of the study, I recommend that you look into the report of scientists and additional materials to it.

Epilogue

The main motivation for this study, scientists call the desire to answer the question - can foam networks show full PBG. The transformation of the edges of dry foam structures into photonic networks showed that they can.



At the moment, the foam is not a particularly studied structure. Of course, there are studies that give good results in terms of amorphous networks, but they were carried out at extremely small sites. How the system will behave with an increase in its size remains unclear.



According to the authors of the study, their work opens up many opportunities for future inventions. Foam is very common in nature and easy to manufacture, which makes this structure very attractive for practical use.



One of the most ambitious options for applying their research, scientists call the Internet. According to the researchers themselves, the transmission of data via optical fiber is not an innovation, but at the destination, the light is still converted to electricity. Materials with photonic band gaps can direct light much more accurately than conventional fiber optic cables, and can serve as optical transistors that perform calculations using light.



No matter how grandiose the plans may be, much work remains to be done. However, neither the complexity of research, nor the complexity of experimentation can defeat the enthusiasm of scientists and their desire to improve the world of technology.



Thank you for your attention, stay curious and have a great weekend everyone, guys! :)



Thank you for staying with us. Do you like our articles? Want to see more interesting materials? Support us by placing an order or recommending it to your friends, cloud VPS for developers from $ 4.99 , a 30% discount for Habr users on the unique entry-level server analog that we invented for you: The whole truth about VPS (KVM) E5-2650 v4 (6 Cores) 10GB DDR4 240GB SSD 1Gbps from $ 20 or how to share a server? (options are available with RAID1 and RAID10, up to 24 cores and up to 40GB DDR4).



Dell R730xd 2 times cheaper? Only we have 2 x Intel TetraDeca-Core Xeon 2x E5-2697v3 2.6GHz 14C 64GB DDR4 4x960GB SSD 1Gbps 100 TV from $ 199 in the Netherlands! Dell R420 - 2x E5-2430 2.2Ghz 6C 128GB DDR3 2x960GB SSD 1Gbps 100TB - from $ 99! Read about How to Build Infrastructure Bldg. class c using Dell R730xd E5-2650 v4 servers costing 9,000 euros for a penny?