Understanding particle physics: 2) a quantum ball on a spring
1. A ball on a spring, the Newtonian version
2. Quantum ball on spring
3. Waves, classic look
4. Waves, the classical equation of motion
5. Quantum waves
6. Fields
7. Particles are quanta
8. How particles interact with fields
The key result of the previous article was that the oscillatory motion of a ball on a spring in prequant physics of Newton and his friends takes the form
Where:
• z - the position of the ball as a function of time t,
• z 0 is the equilibrium position of the ball (where it would have been at rest if it had not oscillated),
• A is the amplitude of oscillations (which we can choose as large or small as we like),
• ν [nu] is the oscillation frequency (depending only on the spring strength K and the mass of the ball M, and independent of A).
In addition, the total energy stored in the oscillation is equal to
By changing A, we can save any amount of energy in oscillation.
In quantum mechanics, everything changes. At first glance (and we don’t need more), only one thing changes - the statement that the amplitude of oscillations can be chosen arbitrarily large or small. It turns out that this is not the case. Accordingly, the energy stored in the oscillation cannot be chosen arbitrarily.
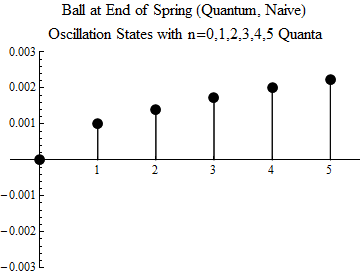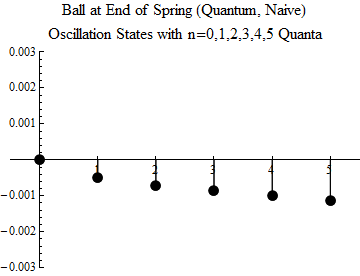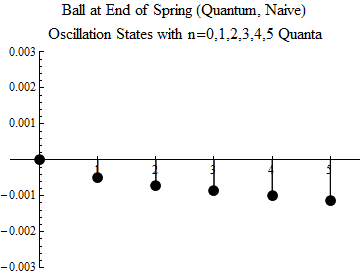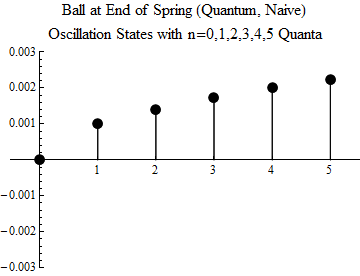
Fig. one
Max Planck, the famous physicist of the early 20th century, discovered that there is something quantum in the Universe and introduced a new constant of nature, which is called the constant Plank, h. Every time you encounter anything in quantum mechanics, you will see h. Quantitatively
- a very small value for ordinary human life. And that's what comes out:
A quantum ball on a spring can oscillate only with amplitudes
Where n is an integer, for example, 0, 1, 2, 1798 or 2 348 979. The oscillations are not arbitrary, but quantized: we can call n the quantum of oscillations. Definition: we say that a sphere oscillating with a quantum n is in the nth excited state. If the quantum is zero, we say that it is in the ground state.
To make you understand what this means, the first five excited states, and the ground state, are shown (quite naively — you should not take the image too seriously) in Fig. 1. The minimum possible oscillation occurs in the state n = 1. This is a quantum of oscillations; there is no quantum share. The ball can not oscillate less, unless it is in a state without fluctuations, when n = 0.
Everything else, at first glance, is the same. But in fact, the history of quantum mechanics is much more complicated! But for now, we can move away from this confusion and use almost 100% true physics.
Why can not we say that the oscillations are quantized based on our experience? Because in everyday systems, quantization is too small. Take a real ball and spring - say, the ball weighs 50 grams, and the frequency of its oscillations - once a second. Then the oscillation for one quantum, n = 1, will correspond to the amplitude
This is a couple of tens of thousands of millionths of a millionth of a meter, or 10 times less than a proton! One quantum of oscillations will not move the ball even a distance of the order of the size of an atomic nucleus! No wonder we see no quantization! If the ball moves a visible distance, it contains a huge number of quanta - and for such large n from our point of view, we can make any A, see fig. 2. We cannot measure A sufficiently accurately to notice such subtle restrictions on its size.

Fig. 2. The amplitude of vibration A for state n. For small n, the individual values of A lie far apart, but for n = 100, the permitted values of A lie so close that it is very difficult to notice the discreteness. In everyday situations, the values of n are so great that it is impossible to discern discreteness.
Note that in particular such values are obtained due to the large mass of the ball. If the ball consisted of 100 iron atoms and had a radius of one thousandth of a millionth of a meter, its minimum amplitude would be one millionth of a meter, that is, it would be a thousand times larger than its radius. And it is large enough so that it can be seen under a microscope. But such a small ball would be exposed to forces operating on an atomic scale, and would oscillate much faster than once a second - and the higher frequency corresponds to small amplitudes. So even with a small ball, it is not easy to notice the quantization of nature.
Now let's take the amplitude quantization, and place it in the formula for oscillation energy, which we mentioned at the beginning of the article, . Substituting in it the allowed values for A, we get an amazing result:
Amazingly simple answer! The energy stored in a quantum ball on a spring (naively) is proportional to n, the number of oscillation quanta, the constant bar h and the oscillation frequency ν. Even more surprising, this simple formula is in fact almost accurate! What does she show right?
• The energy that must be spent on increasing the number of quanta in oscillations by one, (n → n + 1), is h ν.
• In any oscillator encountered in everyday life, the energy of one quantum will be so small that we will never know about its quantization.
Check it out. For a ball with a spring oscillating once a second, one quantum of energy will be equal to 6.6 × 10 -34 J, or 0.000 000 000 000 000 000 000 000 000 000 66 Joules. And Joule - this is the energy that you spend, lifting an apple from the ground to the level of the belt - not so big! So this is an incredibly small amount of energy. Only in small molecules and even smaller systems can the oscillation frequency be so large that quantization of energy can be detected.
It turns out that the formula for energy is not quite correct. By doing these calculations for quantum mechanics, you can find that the correct formula for energy will be:
We often do not need to pay attention to this small shift n by 1/2. However, it is very interesting - it is from him that all the intricacies of quantum mechanics begin. Isn't that curious? Even if there are no oscillation quanta in the oscillator at all, when n = 0, it still contains a small amount of energy. It is called zero-point energy, or zero energy, and is taken from the fundamental jitter, the basic unpredictability that lives in the heart of quantum mechanics. Look at the pic. 3, which is inevitably schematic and inaccurate, tries to demonstrate how jitter is responsible for zero energy. The ball moves randomly, even in the ground state. In the future, we will return to zero energy, because it will lead us to the deepest problems of all physics.

Fig. 3. The fundamental unpredictability of quantum mechanics can be thought of as an occasional jitter that changes the position of the ball. It randomly moves even in the ground state, and also affects the excited ones, although with an increase in n, its effect is not so noticeable. The drawing is sketchy and should not be taken too seriously.
2. Quantum ball on spring
3. Waves, classic look
4. Waves, the classical equation of motion
5. Quantum waves
6. Fields
7. Particles are quanta
8. How particles interact with fields
The key result of the previous article was that the oscillatory motion of a ball on a spring in prequant physics of Newton and his friends takes the form
Where:
• z - the position of the ball as a function of time t,
• z 0 is the equilibrium position of the ball (where it would have been at rest if it had not oscillated),
• A is the amplitude of oscillations (which we can choose as large or small as we like),
• ν [nu] is the oscillation frequency (depending only on the spring strength K and the mass of the ball M, and independent of A).
In addition, the total energy stored in the oscillation is equal to
By changing A, we can save any amount of energy in oscillation.
In quantum mechanics, everything changes. At first glance (and we don’t need more), only one thing changes - the statement that the amplitude of oscillations can be chosen arbitrarily large or small. It turns out that this is not the case. Accordingly, the energy stored in the oscillation cannot be chosen arbitrarily.

Fig. one
Quantization of vibration amplitude
Max Planck, the famous physicist of the early 20th century, discovered that there is something quantum in the Universe and introduced a new constant of nature, which is called the constant Plank, h. Every time you encounter anything in quantum mechanics, you will see h. Quantitatively
- a very small value for ordinary human life. And that's what comes out:
A quantum ball on a spring can oscillate only with amplitudes
Where n is an integer, for example, 0, 1, 2, 1798 or 2 348 979. The oscillations are not arbitrary, but quantized: we can call n the quantum of oscillations. Definition: we say that a sphere oscillating with a quantum n is in the nth excited state. If the quantum is zero, we say that it is in the ground state.
To make you understand what this means, the first five excited states, and the ground state, are shown (quite naively — you should not take the image too seriously) in Fig. 1. The minimum possible oscillation occurs in the state n = 1. This is a quantum of oscillations; there is no quantum share. The ball can not oscillate less, unless it is in a state without fluctuations, when n = 0.
Everything else, at first glance, is the same. But in fact, the history of quantum mechanics is much more complicated! But for now, we can move away from this confusion and use almost 100% true physics.
Why can not we say that the oscillations are quantized based on our experience? Because in everyday systems, quantization is too small. Take a real ball and spring - say, the ball weighs 50 grams, and the frequency of its oscillations - once a second. Then the oscillation for one quantum, n = 1, will correspond to the amplitude
This is a couple of tens of thousands of millionths of a millionth of a meter, or 10 times less than a proton! One quantum of oscillations will not move the ball even a distance of the order of the size of an atomic nucleus! No wonder we see no quantization! If the ball moves a visible distance, it contains a huge number of quanta - and for such large n from our point of view, we can make any A, see fig. 2. We cannot measure A sufficiently accurately to notice such subtle restrictions on its size.

Fig. 2. The amplitude of vibration A for state n. For small n, the individual values of A lie far apart, but for n = 100, the permitted values of A lie so close that it is very difficult to notice the discreteness. In everyday situations, the values of n are so great that it is impossible to discern discreteness.
Note that in particular such values are obtained due to the large mass of the ball. If the ball consisted of 100 iron atoms and had a radius of one thousandth of a millionth of a meter, its minimum amplitude would be one millionth of a meter, that is, it would be a thousand times larger than its radius. And it is large enough so that it can be seen under a microscope. But such a small ball would be exposed to forces operating on an atomic scale, and would oscillate much faster than once a second - and the higher frequency corresponds to small amplitudes. So even with a small ball, it is not easy to notice the quantization of nature.
Oscillation energy quantization
Now let's take the amplitude quantization, and place it in the formula for oscillation energy, which we mentioned at the beginning of the article, . Substituting in it the allowed values for A, we get an amazing result:
Amazingly simple answer! The energy stored in a quantum ball on a spring (naively) is proportional to n, the number of oscillation quanta, the constant bar h and the oscillation frequency ν. Even more surprising, this simple formula is in fact almost accurate! What does she show right?
• The energy that must be spent on increasing the number of quanta in oscillations by one, (n → n + 1), is h ν.
• In any oscillator encountered in everyday life, the energy of one quantum will be so small that we will never know about its quantization.
Check it out. For a ball with a spring oscillating once a second, one quantum of energy will be equal to 6.6 × 10 -34 J, or 0.000 000 000 000 000 000 000 000 000 000 66 Joules. And Joule - this is the energy that you spend, lifting an apple from the ground to the level of the belt - not so big! So this is an incredibly small amount of energy. Only in small molecules and even smaller systems can the oscillation frequency be so large that quantization of energy can be detected.
It turns out that the formula for energy is not quite correct. By doing these calculations for quantum mechanics, you can find that the correct formula for energy will be:
We often do not need to pay attention to this small shift n by 1/2. However, it is very interesting - it is from him that all the intricacies of quantum mechanics begin. Isn't that curious? Even if there are no oscillation quanta in the oscillator at all, when n = 0, it still contains a small amount of energy. It is called zero-point energy, or zero energy, and is taken from the fundamental jitter, the basic unpredictability that lives in the heart of quantum mechanics. Look at the pic. 3, which is inevitably schematic and inaccurate, tries to demonstrate how jitter is responsible for zero energy. The ball moves randomly, even in the ground state. In the future, we will return to zero energy, because it will lead us to the deepest problems of all physics.

Fig. 3. The fundamental unpredictability of quantum mechanics can be thought of as an occasional jitter that changes the position of the ball. It randomly moves even in the ground state, and also affects the excited ones, although with an increase in n, its effect is not so noticeable. The drawing is sketchy and should not be taken too seriously.
All Articles