Beautiful math monsters

UPD: feature graph added.



Like its creator Karl Weierstrass, this monster sprang from nowhere. After spending four years of studying at the university for reveling and fencing, Weierstrass left him empty-handed. In the end, he took up teaching and most of the 1850s was a school teacher in Brownsburg. He was disgusting life in a small Prussian town, he found his existence there alone. The only outlet for him was the mathematical problems on which he worked between lessons. But he had no one to talk about mathematics, and he did not have a technical library for training. Even the results of his work could not leave the limits of Brownberg. Instead of publishing in academic journals, as a university researcher would have done, Weierstrass added them to school brochures, scaring potential students with abstruse equations.



In the end, Weierstrass sent one of his articles to the respected Krell Journal . Despite the fact that the previous articles remained barely noticed, this caused a huge surge of interest. Weierstrass discovered a way to work with a terrible class of equations known as “Abelian functions”. The article provided a brief summary of his methods, but this was enough to convince the mathematicians that the author had a unique talent. Less than a year later, the University of Königsberg gave Weierstrass an honorary doctoral degree, and soon after, the University of Berlin offered him the post of professor. Despite the fact that Weierstrass has done an intellectual analogue of the “from rags to riches” way, many of his old habits have been preserved. He rarely published articles, preferring to share his work with students. But he was of little respect not only for the process of publication: the "sacred cows" of mathematics did not frighten him either.



Soon, Weierstrass set about studying the works of Augustin Louis Cauchy, one of the most prominent mathematicians of the century. In many papers, Cauchy investigated mathematical analysis and rates of change (or "derivatives"). He created the basics of the dictionary of mathematical analysis, identifying the most important concepts on this subject. But when Weierstrass saw his definitions, he found them wordy and vague. They had too many “hand gestures” and few details.



He decided to revise the Cauchy dictionary, replacing prose with logical conditions. The main goal in his early work was to redefine the derivative. To calculate the gradient of the curve at a point, and hence the rate of its change, Isaac Newton initially considered the line passing through this point and the adjacent point of the curve. Then he shifted this neighboring point closer and closer until the slope of the curve became equal to the gradient of the curve. But this concept was difficult to determine mathematically. What indicates the "proximity" of two points to each other?



According to Cauchy’s verbose definition, the gradient “approaches indefinitely a fixed value, so that as a result it differs from it as much as is required.” Weierstrass did not consider that such a description is sufficiently clear. He wanted to create a more practical definition, so he decided to turn the concept into a formula. Instead of manipulating abstract ideas, mathematics should be able to change equations. Working on this, he laid the foundations of his monster.



In that era, mathematics was largely inspired by nature. When Newton developed mathematical analysis, he was primarily inspired by the physical world: the trajectories of the planets, the oscillations of a pendulum, the movement of a falling fruit. Such thinking led to the emergence of geometric intuition regarding mathematical structures. They should have the same meaning as a physical object. As a result, many mathematicians focused on the study of "continuous" functions. They can be perceived as functions that can be drawn without removing the pencil from the paper. Put on the graph the speed of a falling apple depending on the time and it will be a solid line, in which there will be no gaps or sharp jumps. The continuous function was considered to be “natural.”



In the generally accepted opinion then, for any continuous curve, it was possible to find a gradient for any finite number of points. It seemed to correspond to an intuitive understanding: the line may have several uneven pieces, but there are always several parts that are “smooth”. The French physicist and mathematician Andre-Marie Ampere even published proof of this statement. His argument was built on the “intuitively obvious” fact that a continuous curve has parts on which it grows, decreases, or remains flat. This meant that a gradient could be calculated for these areas. Ampere did not think about what would happen when these parts become infinitely small, but he declared that this was not necessary. His approach was general enough to avoid the consideration of " infiniment petits " (infinitely small) elements. Most of the mathematicians of this logic was enough: by the middle of the 19th century, almost every textbook on mathematical analysis cited Ampere's proof.



But in the 1860s, rumors of a strange creature appeared — a mathematical function that contradicted Ampere's theorem. In Germany, the great Bernhard Riemann told his students that he knows a continuous function that does not have smooth parts, and for which it is impossible to calculate the derivative of a function at any point. Riemann did not publish evidence, as did Charles Sellery of the University of Geneva, who wrote that he discovered something “very important and, I think, new,” but hid his work in a folder that became public only after his death for several decades. later. However, if his statements were to be believed, then this would mean a threat to the very foundations of the emerging mathematical analysis. This being threatened to destroy the happy friendship between mathematical theory and the physical observations on which it was based. Mathematical analysis has always been the language of planets and stars, but how can nature be a reliable source of inspiration if there are mathematical functions that contradict its basic essence?



The monster was finally born in 1872, when Karl Weierstrass announced that he had found a function that is continuous but not smooth at all points. He created it by adding together an infinitely long series of cosine functions:







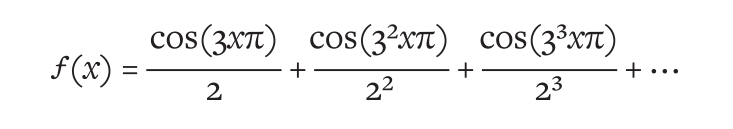
As a function, it was ugly and disgusting. It was not even clear how it would look on the chart. But Weierstrass didn't care. His proof did not consist of forms, but of equations, and that was what made his statement so powerful. He not only created the monster, but also built it on iron logic. He took his own new strict definition of the derivative and proved that it is impossible to calculate it for this new function.



The result brought the mathematical community into a state of shock. The French mathematician Emile Picard said that if Newton knew about such functions, he would not have created a mathematical analysis. Instead of carrying ideas about the physics of nature, he would be stuck in an attempt to get through the hard mathematical obstacles. The monster began to shake and previous research. The results, which seemed "proven", cracked at the seams. Ampere used the lengthy definitions chosen by Cauchy to prove his smoothness theorem. Now his evidence began to crumble. Uncertain notions of the past were powerless against the monster. Even worse was the fact that now it has become unobvious, what the mathematical proof consists of. The intuitive geometric arguments of the past two centuries have become useless. When mathematicians tried to drive the monster away, it remained adamant. With one bizarre equation, Weierstrass showed that physical intuition was not a reliable basis for constructing mathematical theories.







Authoritative mathematicians tried to dismiss the result, arguing that he was ugly and unnecessary. They feared that primers and troublemakers would bring havoc into their favorite field of knowledge. At the Sorbonne, Charles Hermite wrote: "I turn away in disgust and horror from the unfortunate foulness of functions that have no derivatives." Henri Poincaré, who first called such functions monsters, called Weierstrass’s work “an insult to common sense.” He argued that such functions are a cheeky distraction from the subject matter.



“They were invented to show the fallacy of the reasoning of our predecessors,” he said. "And besides this, we can not take anything from them."



Many of the “old guards” wanted to leave the Weierstrass monster in the backyard of mathematics. It was also hampered by the fact that no one could imagine the look of an animal with which they met - only after the invention of computers did it become possible to create its schedule. His incomprehensible form made it difficult for the mathematical community to realize how such a function can exist. In addition, the Weierstrass proof style was unknown to many mathematicians. His proof contained dozens of logical steps and extended over several pages. The chain of reasoning was barely perceptible and required serious technical knowledge, and in the real world there were no analogs that would help in understanding. Instinct urged to avoid this proof.







But monsters have the custom to make their own way. In fact, many concepts that seem today obvious, even necessary, were once monsters. For centuries, mathematics has rejected negative numbers. The ancient Greeks, who mostly worked with geometry, did not see any need for them. The same is true for medieval academicians who adopted the ideas of the Greeks. The shadow of this monster sometimes flashes today, for example, in questions of a child asking why when multiplying two negative numbers we get a positive one. But in general, we have tamed this beast: no one wants to expel him again.



The same thing happened with the Weierstrass monster - it began to receive a vocation. In 1904, Albert Einstein introduced physicists to the idea of ​​"Brownian motion": he said that particles in a liquid follow random paths, because liquid molecules constantly repel them. Collisions are so frequent (more than 10 ²¹ per second) that no matter how good the microscope or carefully observed, the trajectories will never be smooth. From a practical point of view, it is impossible for them to find a derivative. If researchers want to work with such tasks, they will have to face the Weierstrass monster. That is exactly what Einstein did. His theory was that infinitely broken functions were used in the Brownian motion. This became an important precedent: since then, physicists have used non-smooth functions as an approximation to the Brownian motion.



When it became apparent that the so-called “Weierstrass functions” were actually quite useful, scientists began to develop ways to work elegantly with nonsmooth functions. Instead of analyzing the path of an individual particle in a liquid, they began to consider the average behavior of a multitude of particles. How far can they move? When can they reach a given point? Outside the area of ​​study of the Brownian movement, mathematicians also began to rethink the basic tools of mathematical analysis. The rate of change was always determined relative to distances, and the area under the curve was measured geometrically. But when the functions were not smooth, then such ideas did not make sense.



Kiyoshi Ito of Tokyo University found a way around the problem by approaching it in terms of probabilities. It was an unorthodal, if not risky, tactic: in the 1940s hardly anyone considered probability theory to be a serious area. However, Ito insisted on his. He approached functions as random processes and translated Weierstrass definitions into a new language based on probabilities. He stated that two random processes are “close” to each other if their expected results are the same. He introduced a method of working with a mathematical function, depending on the amount of non-smoothness, as in Brownian motion, instead of a more traditional variable, for example, distance. Using his new methods, he derived the “Ito lemma” to calculate the change of such a function with time.



By the 1970s, his work unfolded in a completely new area of ​​mathematics, called stochastic calculus (mathematicians like to call everything related to randomness "stochastic"). As in the analysis itself, a completely new set of tools and theorems appeared in it. Today, stochastic calculus is used to study all sorts of phenomena, from neurons working in the brain to the spread of diseases in the population. It also became the basis of financial mathematics, in which it helps banks evaluate the value of options. It can take into account the uneven behavior of the exchange rate, and therefore, shows how much the value of the option changes over time. The resulting equation, which is known as the Black-Scholes formula, is now used in all trading floors of the world. However, Ito was always confused by the praise of bankers. He was a theoretical mathematician, and did not expect that his work would become famous thanks to practical application.



Weierstrass monster shaken up and the principles of geometry. At the end of the 19th century, the Swedish mathematician Helge von Koch became interested in the idea of ​​non-smooth functions, but wanted to study their form. He set about creating a form (and not a function) that would never be smooth anywhere, thus showing that the same monsters are hiding in algebra with geometry. Although he could not draw the Weierstrass function, he managed to capture her close relative. Working on this task in the process of constantly searching for temporary jobs as an intern teacher, in 1904, von Koch discovered his being. He took an equilateral triangle, then added three smaller triangles on each side, and so on to infinity. The resulting geometric shape was continuous, but had no derivatives. Due to its outstanding appearance, the figure quickly became known as the Koch snowflake.



Koch was able to extend the power of the Weierstrass monster beyond the world of equations and functions. But as a result of his work there was something else that deserved attention. Upon closer examination, it turned out that his snowflake had a curious self-similarity: increase one part of the snowflake, and it will look just like a bigger figure. After many years, it became apparent that the Weierstrass function has the same property.



Over time, this self-similarity began to manifest itself in all sorts of phenomena. To popularize the idea of ​​"fractal" objects in the 1980s, the fundamental work of Benoit Mandelbrot was required. Such objects have forms that are repeated on a smaller and smaller scale. Coastlines, clouds, plants, blood vessels - mathematicians found that fractals are ubiquitous in nature. Like the Koch snowflake, none of them were smooth. Yes, and how would they be smooth? If the figure has smooth parts, then the pattern will disappear with a sufficient magnification. As Koch discovered, the simplest way to get a non-smooth figure is to create a fractal object. Perhaps the work of Weierstrass was inevitably to send mathematicians towards the study of self-similar patterns, introducing the researchers to the world of refined, beautiful structures.



The Weierstrass monster continues its work to this day. The Navier-Stokes equations describe the motion of a fluid and underlie the modern dynamics of fluids and aerodynamics that control everything — from aircraft design to weather forecasting. However, despite the fact that they were first created in the 1840s, mathematicians still do not know whether they can always be solved. In 2000, the Clay Mathematical Institute offered a $ 1 million prize to anyone who would prove that these equations always have smooth solutions — or find an example of the opposite. This task is considered to be one of the six most important outstanding problems of mathematics, because despite the wide use of the Navier-Stokes equations, mathematicians do not know whether these equations always give physically reliable results. The prize of 1 million dollars is still not claimed by anyone. In many ways, this is a price for the head, which provokes mathematicians to hunt for dangerous monsters.



In various areas: from fluid dynamics to financial sector, creatures like Weierstrass have questioned our views on the relationship between mathematics and the natural world. The mathematicians who lived in Weierstrass’s time believed that the most useful mathematics was inspired by nature, and that Weierstrass’s work did not fit this definition. But the stochastic calculus and Mandelbrot fractals proved them wrong. It turned out that in the real world - in the chaotic, complex real world - the monsters are hiding everywhere. As Mandelbrot said, "nature played a joke on mathematicians." Even Weierstrass himself was the victim of this stunt. , , . , , , , . , . , . , - .



: — , .